 @ 2014-02-16 4:35 PM (#14337 - in reply to #14334) (#14337) Top
@ 2014-02-16 4:35 PM (#14337 - in reply to #14334) (#14337) Top
Posts: 1869








Country : India
debmohanty posted @ 2014-02-16 4:35 PM
Can someone please rephrase the instructions for Mini Coral?
So strictly 2 of 4 corners must be shaded in every cell. All white continuous regions must touch the border.
No 2x2 corners can be shaded. And the numbers... shouldn't the 1s be 2s? Since the big white area contains two 7s and it is 14 cells big.
What am I misunderstanding?
- You have to colour 2 of the four corners of each cell, but they must be adjacent. (In Corral you can never have a checkerboard pattern, so that is pretty much forced)
- Nowhere a 2x2 area of four corners can be coloured.
- The numbers indicate the amount of cells (or half cells), not the amount of corners. There's 2 corners per cell white, but they count as a single cell. So the 7s both indicate the same area of 7 cells (or 14 corners). The 1s indicate 1 cell (or 2 corners). Just like in for example Fillomino multiple numbers can appear in the same area as long as they both indicate the size of the area in cells (not corners). So if you want you can see each clue as its double and count the number of corners as all white corners per cell are connected.
In the original versions (see puzzles at LMD), Berni had used the number of corners/quarters. But for Marathon, he suggested that we divide the clues by 2 so that each clue shows the numbers of connected cells. I agreed that it is a better representation.
 @ 2014-02-16 5:00 PM (#14338 - in reply to #14336) (#14338) Top
@ 2014-02-16 5:00 PM (#14338 - in reply to #14336) (#14338) TopPosts: 70



Country : France
Puzlifouk posted @ 2014-02-16 5:00 PM
 @ 2014-02-16 7:21 PM (#14339 - in reply to #14209) (#14339) Top
@ 2014-02-16 7:21 PM (#14339 - in reply to #14209) (#14339) TopPosts: 70



Country : France
Puzlifouk posted @ 2014-02-16 7:21 PM
I mean: is it possible to have the numbers 2 and 3 in the same region whose size is 2 or 3 (and why not a region whose size is 2 with numbers 2 and 2) or the two numbers in a same region are they necessarily separated by at least 2 units ?
 @ 2014-02-16 7:23 PM (#14340 - in reply to #14339) (#14340) Top
@ 2014-02-16 7:23 PM (#14340 - in reply to #14339) (#14340) TopPosts: 3574





Country : India
Administrator posted @ 2014-02-16 7:23 PM
Concerning ARAF, does "between" refer to a strict inequality, or not.
I mean: is it possible to have the numbers 2 and 3 in the same region whose size is 2 or 3 (and why not a region whose size is 2 with numbers 2 and 2) or the two numbers in a same region are they necessarily separated by at least 2 units ?
 @ 2014-02-17 12:20 PM (#14344 - in reply to #14209) (#14344) Top
@ 2014-02-17 12:20 PM (#14344 - in reply to #14209) (#14344) TopPara's Puzzle Site
Para's Puzzle Site posted @ 2014-02-17 12:20 PM
Link to original post : Tutorial: Turning Fences
This week the LMI Puzzle Marathon will start. It will run from February 21st till March 2nd. For this event I have written a Turning Fences puzzle. To prepare people a bit for a more unknown genre, I will write a tutorial about the logic behind the genre. It won't be complete, but it should be enough to tackle the upcoming puzzle. In the next days there will also be a few practise puzzles. You can also practise some of the puzzles already on my blog, but most of these are logically harder than the puzzle in the actual test. For those who happen to own Akil Oyunlari Issue #75, there are also 4 of these puzzles by my hand in there. They are logically a bit more friendly.Rules
Draw a single closed loop by connecting dots horizontally and vertically. The numbers in the grid indicate the amount of turns taken on the four dots around it.
Basics
The puzzle type somewhat looks like the standard Slitherlink puzzles and has some similarities in the layout and the way you have to avoid multiple loops. But that's where the comparison in solving pretty much stops. The solving of a Turning Fences puzzle pretty much always starts on the edge of the grid. This is because knowing that a turn finds place on a dot, still leaves four ways to draw that turn, but on the edge this is reduced to two ways. So to start Turning Fences puzzles you should direct your focus to the edges and corners of the grid.
It is important to keep good track of which dots are turns and which dots are not turns in this puzzle type, even if you can't actually deduce the way the loop goes through the loop. Knowing that one dot can't be a turn or has to be a turn, can determine the other dots around a number and give you lines and/or crosses.
For this tutorial I will be indicating dots that have turns with green and dots that don't have turns with red.
If a dot on the edge has a turn, you can always draw a line away from the edge. If a dot on the edge has no turn, you can cross out this line running away from the edge.
If a line reaches a dot without a turn it will always go straight, so can draw the line through the dot and cross out the sides. If a line reaches a dot with a turn, the line will have to run, so you can cross out the segment going straight.
If there is a cross next to a dot with a turn, then a line segment can be drawn in the opposite direction. If a cross is next to a dot without a turn, then a cross can be drawn in the opposite direction.
That is the basic solving technique of this puzzle type.
Numbers on the edge of the grid
As mentioned before, the edge of the grid is the starting point of any Turning Fences puzzle. So what can we do with numbers that are on the edge of the grid.
The number 0
The number 0 on the edge of the grid is pretty simple. You can draw four crosses as the loop always have to turn when leaving the edge of the grid. The same then goes on the dot one row off the edge.
The number 4
The number 4 on the edge has 3 different ways of being drawn. These options all have 2 lines and 2 crosses in common. So you can draw these at the start.
The number 2
This is where the logic gets a bit more complicated. There are too many options to draw out on how the turns can behave. There are already 6 ways to distribute the places where the turns are located.
But there are some deductions that can be done with a 2 on the edge. The two indicated segments running away from the 2 are always both a line segment or always both a cross.
The number 1 and 3
I am doing these together as the logic on the edge for both is the same. Just like the number 2, there are too many ways this number can behave to draw any direct deductions on its own. But just like the number 2, there is some generalised logic to be learned from these numbers.
With the number 1 and 3, the two indicated line segments always have one segment being a cross and one segment being a line.
As you can see I indicated that the two dots under the cross in the 1 can't have a turn and under the 3 both have a turn. This lets you draw the extra line and cross in the image.
Practise puzzle #1
Here is a first practise puzzle to show how the above can be applied.
Now we can look at the numbers to the right of the 0. As we know that one of the segments leading away of the 1 is a cross, the other must be a line. Now we can look at the 2 and deduce that the other segment leading away from the 2 is also a line. Then we look at the 3, where we know the other segment leading away from the 3 must be a cross. We also know where two of the turns around this 3 now go and we can mark these as turns.
We can do a similar thing with the numbers to the left of the 4, leading to the given lines, crosses and no turns.
Now we can look at some of the other numbers. We can see that both 3s on the right already have one dot marked as not being a turn. This means that for both the remaining three dots must be turns. Now we have both 2s on the left with two turns marked, so we know that the other two dots must not be turns. Now we know how those dots behave, we can draw the given lines and crosses.
When we look at the right, we can see that the top 1 already has one dot marked as being a turn, so we know the other dots can't be turns. This means we can draw a number of crosses and lines around the 1. If we look at the 1 below, it has one cross leading away from it, so the other must be a line. IF we then look at the 2, we can see it has one line leading away from it, so the other segment must also be a line. The same now goes for the 2 below that.
Now we can draw some more loop if we look at the bottom right. The lines around the 4 must escape to the right and left. To the left it has to connect to the line between the 2 and 3.
Now you can look at the left and see that the end in the bottom has to go up and the two end in top have to connect to eachother.
When you then look to the right top, you can see this end has to escape to the right and downwards.
Now we come to a bit trickier step that we haven't discussed before. But it should be clear anyway.
If we look at the 1 in the middle, we can see that the right lower dot can't be a turn. Because if it were a turn, the line would have to travel up, but then the right top dot would also be a turn. That would mean we have two turns around a 1 and that is not allowed. So we know the right lower dot is not a turn and the line has to go straight.
This is one of the more important observations you can make in the later stages of a solve of a Turning Fences puzzle, to avoid getting more turns then allowed around a number.
There is also a similar rule for a 1 and 3 in the centre. The rule is that there is always an odd number of horizontal line segments leading to a 1 or 3. Again the same goes for the number of vertical line segments. Below some examples of that.
There are a lot of ways these patterns can be hidden. It is therefore important to remember the logic behind these patterns. Try to notice when a pair of dots can only have one dot, or when a pair of dots needs to have at least one dot. And then see if this interacts with the numbers around them.
The distant 0
A 0 can lead to interactions from a distance. This occurs when a line is forced through them, but you aren't sure which one yet. This may sound a bit confusing, but I will explain.
As mentioned before, a 1 or 3 on the edge must have one line leading away from it and one cross. If either of these lines would reach a 0, then they both would have to go straight. This means that the line can't go through the 0 in the different direction as seen below. This leads to a number of crosses that can be drawn.
Let's now start and look in the left top. We can see from the corner 3-1, we have to go straight at the top and the other dot must be a turn. We can them figure out how the lines go around the 1-3 pair just below it.
Now we look at the 3-1 pair in column four. The left bottom dot can't be a turn, because then the right bottom dot would also be a turn and there are too many turns. So that one has to be a no-turn. We can fill out a number of lines and crosses again now.
Now when we look at the 1 in row 4, we can see it has to turn in the left top dot as otherwise we would create a loop. Thus the other three dots are no-turns. Now we have two no-turns around the 2 in row four, so the other two dots must be turns. We can draw a bit more of the loop to the right.
Now we look at the 1 in row six. The left top dot can't be a turn as then we would have two turns around the 1, so the line goes straight down. The right top can't be a turn as it would create an extra loop, so it goes down straight. Now the left bottom dot can't be a turn as it would either create an extra loop or two turns around the 1, so the line goes straight down again. This means that the right bottom dot has to be a turn.
Around the 2 in row six, we now have to avoid creating an extra loop. To do that the right top dot has to be a turn. This leads to more line segments using the 4 and surrounding numbers.
We can now draw some simple line segments using the 3 in row seven as the left bottom dot has to be a turn, now the left top dot is a no-turn. We can also apply some edge reasoning with the 1-2 pair in row ten.
Now we come to the hardest part of the puzzle. this really uses the logic we learned about numbers in the middle of the grid. From the edge logic for 2s we know that both the purple circles have to be a line segment or both have to be a cross. This means there's always an even number of line segments there. If we now look at 1 in column eight, we can deduce the fourth vertical segment. We know the 1 always has an odd number of vertical line segments going to it. As the top two vertical line segments always have an even number of line segments, we know that the bottom two vertical segments always have to have an odd number of line segments to create an odd total. As we already have one cross, we know that the one line segment has to be a line.
We can employ the same logic with the yellow circles and the 3 in row eight.
Now we can finish filling out the 3 in row eight as we know the right top dot can't be a turn. The whole bottom left can now be filled out by avoiding creating an extra loop. We can also fill out a little bit of loop from the 3 in row seven.
Now with a bit of edge number logic with the 2-3 pair in row ten, we can fill out a lot of the right edge.
A way to continue now, is to count the number of ends in the bottom right corner. We can see there are seven ends there. as we always need en even number of ends to create a loop, we need an eighth loop end. This forces the line segment between the two 4s in column five to go down.
Now a way to fill out this bit is to count the horizontal segments around the second 2 in row nine. We have two horizontal line segments and one cross. As the total of line segments has to be even, the fourth segment has to be a cross. Now the bottom fills out simply.
Now to finish the top right of the grid. We can start at the 4-3 pair in column six. The loop there only has one way to go. This then force the left bottom dot of the 1 in row one to be a turn. So the rest are no-turns. Now the 2 in row one has two no-turn dots, so the other two dots need to be turns as well. And we can draw a whole bit of loop again.
The last bit can easily be solve with some edge number logic on the 3-2 pair in column ten. And finally we have to connect the last two ends around the 1, so it only creates one turn.
Posted in LMI, Naoki, Turning Fences, Tutorial
 @ 2014-02-17 12:26 PM (#14345 - in reply to #14344) (#14345) Top
@ 2014-02-17 12:26 PM (#14345 - in reply to #14344) (#14345) Top
Posts: 1869








Country : India
debmohanty posted @ 2014-02-17 12:26 PM
Link to original post : Tutorial: Turning Fences
I just wanted to highlight the excellent material prepared by Bram for Turning Fences. (Yes, he is the author of the type in Marathon). Don't miss the post - it really helps solving the slitherlink-like-looking-but-very-different Turning Fence. @ 2014-02-17 5:03 PM (#14346 - in reply to #14337) (#14346) Top
@ 2014-02-17 5:03 PM (#14346 - in reply to #14337) (#14346) TopPosts: 1
berni posted @ 2014-02-17 5:03 PM
In the original versions (see puzzles at LMD), Berni had used the number of corners/quarters. But for Marathon, he suggested that we divide the clues by 2 so that each clue shows the numbers of connected cells. I agreed that it is a better representation.
To avoid confusion I now halved all numbers at LMD too (and adjusted the instructions accordingly).
 @ 2014-02-17 5:34 PM (#14347 - in reply to #14209) (#14347) Top
@ 2014-02-17 5:34 PM (#14347 - in reply to #14209) (#14347) TopPosts: 74



Country : South Korea
Joo M.Y posted @ 2014-02-17 5:34 PM
 @ 2014-02-18 8:31 PM (#14351 - in reply to #14209) (#14351) Top
@ 2014-02-18 8:31 PM (#14351 - in reply to #14209) (#14351) TopPosts: 114

Country : India
devarajand posted @ 2014-02-18 8:31 PM
 @ 2014-02-18 11:25 PM (#14353 - in reply to #14209) (#14353) Top
@ 2014-02-18 11:25 PM (#14353 - in reply to #14209) (#14353) TopPosts: 171




Country : Germany
rob posted @ 2014-02-18 11:25 PM
I wouldn't say any of those are particularly easy though.
 @ 2014-02-18 11:31 PM (#14354 - in reply to #14209) (#14354) Top
@ 2014-02-18 11:31 PM (#14354 - in reply to #14209) (#14354) TopPosts: 123


Country : India
macherlakumar posted @ 2014-02-18 11:31 PM
Statue Park:
http://mellowmelon.wordpress.com/category/puzzle-types/statue-park/
2 years back Palmer has released a Statue Park pack pdf, it can be found at http://mellowmelon.files.wordpress.com/2012/03/pack02statuepark_v2....
http://www.gmpuzzles.com/blog/category/statue-park/
Marathon Yajilin and Heyawake:
http://logicmastersindia.com/lmitests/dl.asp?attachmentid=162&view=...
http://logicmastersindia.com/lmitests/?test=M201010P1
Araf:
http://logicmastersindia.com/lmitests/?test=M201303P
http://yureklis.wordpress.com/2012/06/06/puzzle-15-araf/
http://yureklis.wordpress.com/2012/06/05/araf/
Nanro:
http://logicmastersindia.com/lmitests/?test=M201303P
http://www.gmpuzzles.com/blog/category/nanro/
http://prasannaseshadri.files.wordpress.com/2013/04/nanro2.png
http://prasannaseshadri.files.wordpress.com/2013/04/nanro3.png
http://prasannaseshadri.files.wordpress.com/2013/06/nanro.png
Yajilin:
http://prasannaseshadri.wordpress.com/category/yajilin/
http://www.gmpuzzles.com/blog/category/yajilin/
http://puzzleparasite.blogspot.in/search/label/Yajilin
Regards,
Ravi
 @ 2014-02-19 7:12 PM (#14359 - in reply to #14209) (#14359) Top
@ 2014-02-19 7:12 PM (#14359 - in reply to #14209) (#14359) TopPosts: 74



Country : South Korea
Joo M.Y posted @ 2014-02-19 7:12 PM
http://www.logic-masters.de/Raetselportal/Suche/erweitert.php?tag=M...
 @ 2014-02-19 8:01 PM (#14360 - in reply to #14209) (#14360) Top
@ 2014-02-19 8:01 PM (#14360 - in reply to #14209) (#14360) TopPosts: 114

Country : India
devarajand posted @ 2014-02-19 8:01 PM
 @ 2014-02-19 10:03 PM (#14361 - in reply to #14209) (#14361) Top
@ 2014-02-19 10:03 PM (#14361 - in reply to #14209) (#14361) TopPosts: 171




Country : Germany
rob posted @ 2014-02-19 10:03 PM
 @ 2014-02-19 10:54 PM (#14364 - in reply to #14360) (#14364) Top
@ 2014-02-19 10:54 PM (#14364 - in reply to #14360) (#14364) TopPosts: 669





Country : India
swaroop2011 posted @ 2014-02-19 10:54 PM
Thanks for practice puzzles. But I am unable attempt Araf. Any basics to solve it.
I don't know about particular tricks except that
1) if two consecutive numbers are adjacent - then draw a bar between them
2) Two numbers connected should at least have difference of two
These are pretty much from Rules of ARAF.
Others things you can check out is as the size of region has to be between those two numbers, so see if any closed or forced regions are formed, which makes size of region not falling between the two.
I don't know whether i explained clearly, but if you start an puzzle and got stuck then put that here, so we can help out to direct that puzzle. And then may be you can figure out some tricks on your own.
:)
 @ 2014-02-20 7:35 PM (#14369 - in reply to #14360) (#14369) Top
@ 2014-02-20 7:35 PM (#14369 - in reply to #14360) (#14369) TopPosts: 3
Country : Japan
nyoroppyi posted @ 2014-02-20 7:35 PM
(araf-practice.png)
Attachments
----------------
 araf-practice.png (5KB - 5 downloads)
araf-practice.png (5KB - 5 downloads) @ 2014-02-20 9:29 PM (#14370 - in reply to #14209) (#14370) Top
@ 2014-02-20 9:29 PM (#14370 - in reply to #14209) (#14370) TopPosts: 3574
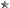





Country : India
Administrator posted @ 2014-02-20 9:29 PM
Please note, this is just an informative post, and is not indented to encourage you to solve on paint.
 @ 2014-02-20 9:36 PM (#14371 - in reply to #14209) (#14371) Top
@ 2014-02-20 9:36 PM (#14371 - in reply to #14209) (#14371) TopPosts: 3574





Country : India
Administrator posted @ 2014-02-20 9:36 PM
Submission page now has puzzle details
Submission page now has details about each puzzle (size / relative difficulty / author).
The puzzles are sorted based on relative difficulty (i.e. easiest puzzle at top & most difficult puzzle at the bottom). Puzzles of similar difficulty are sorted by familiarity. As it happens in many cases, difficulty is a matter of perception and preference. So your experience may differ.
 @ 2014-02-20 10:54 PM (#14373 - in reply to #14209) (#14373) Top
@ 2014-02-20 10:54 PM (#14373 - in reply to #14209) (#14373) TopPosts: 3574





Country : India
Administrator posted @ 2014-02-20 10:54 PM
Test starts 6 hours earlier than planned
Because of administrative constraints, Puzzle Marathon starts 6 hours earlier than planned. Test end time does not change. @ 2014-02-21 8:29 AM (#14375 - in reply to #14209) (#14375) Top
@ 2014-02-21 8:29 AM (#14375 - in reply to #14209) (#14375) TopPosts: 315



Country : The Netherlands
Para posted @ 2014-02-21 8:29 AM

 @ 2014-02-21 8:53 AM (#14376 - in reply to #14209) (#14376) Top
@ 2014-02-21 8:53 AM (#14376 - in reply to #14209) (#14376) TopPosts: 148



Country : France
auroux posted @ 2014-02-21 8:53 AM
 @ 2014-02-21 9:57 AM (#14379 - in reply to #14209) (#14379) Top
@ 2014-02-21 9:57 AM (#14379 - in reply to #14209) (#14379) Top
Posts: 1869








Country : India
debmohanty posted @ 2014-02-21 9:57 AM
 @ 2014-02-21 11:40 AM (#14381 - in reply to #14209) (#14381) Top
@ 2014-02-21 11:40 AM (#14381 - in reply to #14209) (#14381) TopPosts: 2
Country : United States
razapor posted @ 2014-02-21 11:40 AM
Thank You.
Maybe I'm counting wrong.
 @ 2014-02-21 11:46 AM (#14382 - in reply to #14381) (#14382) Top
@ 2014-02-21 11:46 AM (#14382 - in reply to #14381) (#14382) Top
Posts: 1869








Country : India
debmohanty posted @ 2014-02-21 11:46 AM
In Scrabble ....
We expect number of letters.
if you are counting number of letters, and still getting wrong mark, can I ask you to send your solution (a photo) to logicmasteradmin@gmail.com ?
Edited by debmohanty 2014-02-21 11:47 AM
 @ 2014-02-21 12:17 PM (#14383 - in reply to #14382) (#14383) Top
@ 2014-02-21 12:17 PM (#14383 - in reply to #14382) (#14383) TopPosts: 3574





Country : India
Administrator posted @ 2014-02-21 12:17 PM
Score page
Link to score page : http://logicmastersindia.com/2014/02P/score.aspPlease note that, the score page only lists puzzles that you have completed.